The general aim of this project is to study the Renaissance developments of the notion of praxis in geometry and, more specifically, the status of practical geometry and its relation with theoretical and applied geometrical knowledge in the sixteenth century.
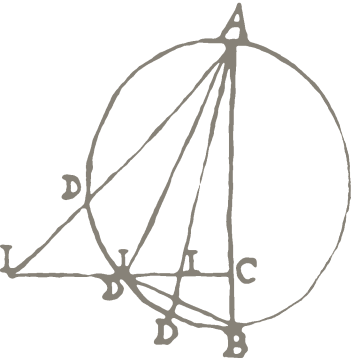
The various treatises published on practical geometry during the sixteenth century (in Italy, France, Spain, Germany and England), which were written by mathematicians of different institutional and cultural contexts and which sometimes greatly differ from each other with respect to the type of teaching they provide (depending on how much they insist on the concrete applications of geometry or, on the contrary, on how much they present practical geometry as an extension of theoretical geometry), reveal the difficulty there is in defining the limits between theoretical and practical knowledge, as well as between practical and applied knowledge in this tradition. In considering these sixteenth-century treatises, the main goal of the project is to shed light on the multiformity and underlying specificities of Renaissance practical geometry, but also, in a more general perspective, to bring forth new elements regarding the transformation of the epistemological, institutional and cultural status of practical knowledge in Early modern Europe, particularly in learned contexts.
Considering a few representative cases, these treatises will be compared according to:
- the context in which they were written;
- the social and intellectual background of their authors;
- the general definition of practical geometry that is given in the prefaces or prologues;
- the intention of the treatises and the aimed readership;
- the type of propositions they contain and their style of teaching, in particular whether these provide a teaching on measuring instruments and their concrete applications and whether these contain propositions derived from theoretical geometry.