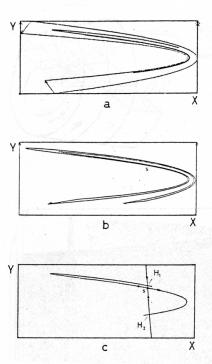
Figs. 1 (above) and 2 are part of a series in which alternating computer images and hand drawn images show different views of the chaotic phase-space attractor that was subsequently given the author’s name (the “Rössler attractor”). The spiral in fig. 2 describes a one-dimensional movement and its folding at the saddle point (S), as shown in fig. 1c. Mathematically speaking, both images depict the same actual situation: one as a numerical approximation; the other as a sketch. Their difference, however, is striking. While the drawn curves are arranged as a kind of bulging flow, the computer image resembles a sharp hairpin bend. The formal distinctions as well as the mutual references regarding content in these two possible modes of representations mark a starting point of the current project.
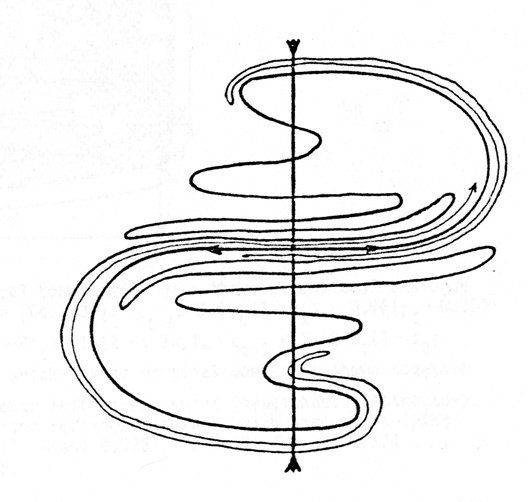
The emergence of a theory of complex dynamics in the 1970s would not have been possible without both analog and digital computer technology as instruments of experimental visualization. Nevertheless, the pencil did not function merely as a supplementary tool of investigation but played a pivotal role in the formation of theories in this field. It was not in spite of but rather because of the emergence of computer generated images—this is my hypothesis—that the pencil became an indispensable tool in the process of extracting a theoretical idea from the bulk of visualized data. In many cases it is the drawn line that enables the passage from experimentally generated images to a concept. This specific function of drawing is exemplified in the working method of the late French mathematician and eminent specialist in this branch of complex dynamics, Adrien Douady.
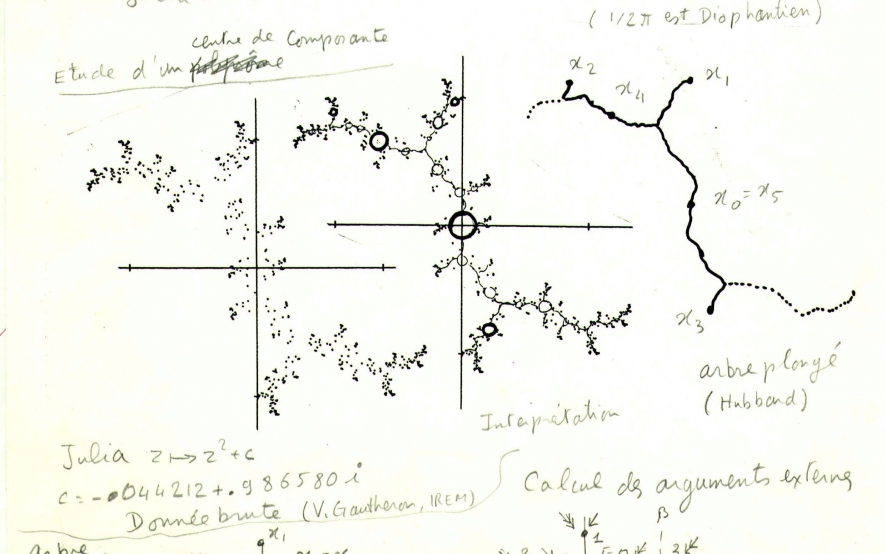
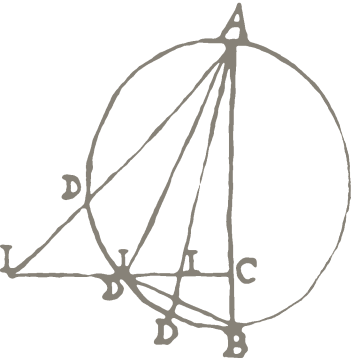
Fig. 3 shows an example of how the raw data (“donnée brute”) of a Julia-Set is transformed in three steps into a drawing – the drawing itself subsequently became the object of investigation (“Hubbard tree”).
In contrast, Otto Rössler’s use of drawing seems to be far more radical. In some cases he even considers it to play a primal role in the computer-generated visualization. According to Rössler, a dynamical chaotic shape (or “Gestalt” as he calls it) has to be initially, “forced onto the paper, a process that can be compared to catching prey. When you are doing a drawing on paper, at some point something snaps into place in an almost audible way.” “force – snapping – death bite” (fig. 4): Rössler uses dramatic and violent terms to describe the moment of finding the form. Only a process of violent taming creates the conditions for a reconciliation between material and analytical mind. After the adequate form has been found by means of drawing, subsequent analytical reasoning proves to be trivial and simple (“Trivialismus,” fig. 4).
Therefore, the scientist’s visual practice will also be analyzed from the viewpoint of a theory of drawing in a more traditionally art historical perspective. Hence, the multifaceted mannerist term disegno will be applied tentatively to different types of mathematical computer images and their interaction with drawings made by hand. Rössler, himself a professed critic of the digital image, used paper and pencil in an accentuated physical and experimental way when developing his concepts on chaos and hyperchaos. He felt that the fingers’ contact with any materiality and the pencil’s abrasiveness on the paper function as material thresholds for thought (“Denkschwellen”) that, in a performative way, can have a retroactive effect on creative ideas, whether artistic or scientific. In the process of drawing, thoughts can crystallize that defy the control of analytical intelligibility since their shapes relate to a realm of intuition beyond calculus. Accordingly, obtaining knowledge—and this also holds for mathematical knowledge—must be considered as a process that is dependent on both the materials and the media of representation.
The analysis of the interdependency of gestural, material, and calculated images in Otto Rössler’s work was complemented and extended by results from research in the private visual archives of Benoît Mandelbrot (Harvard, IBM), John Hubbard (Cornell), and others.